Question about Resultant of gravitational force?

The gravitational force between the Earth and the Moon is strongest at the times of full moon and new moon. During the full moon and new moon phases, the Sun, Earth, and Moon are in alignment, with the Moon being on the opposite side of the Earth from the Sun during a full moon, and on the same side as the Sun during a new moon. This alignment causes the gravitational forces between the Earth and the Moon to reinforce each other, making the tides stronger than they are during other phases of the Moon.

It's worth noting though that the difference in the strength of gravitational forces between the Earth and the Moon during different phases is quite small and not easily noticeable in everyday life.

Referring to image,
New Moon at 1, and Full moon at 5, how can full moon be one of the strongest in resultant of gravitational force on Earth?
Do you have any suggestions?
Thank you very much for any suggestions (^v^)

 

Post Merge: 2 months ago

During full moon, Moon's gravitational force is pulling Earth away from Sun.
Should the resultant of gravitational force be the weakest location on Earth?

Post Merge: 2 months ago

Could you please list out the following resultant gravitational force among Sun and Moon on Earth from strongest to weakest orders?



1) New Moon
2) Waxing Crescent
3) First Quarter
4) Waxing Gibbous
5) Full Moon
6) Waning Gibbous
7) Third Quarter
8) Waning Crescent

Do you have any suggestions?
Thank you very much for any suggestions (^v^)

The tides are created by the gravitational attraction of Moon and Sun. But because the Moon is so close, the Moon gravitation is far more important (about 1560 times more).
Why is tide larger at new Moon or full Moon? That's because then, the Sun and Moon are on the same line and their force is combined. When the Moon is new, it is on the same side of the Earth as Sun is. When is full Moon, the Moon is exactly opposed to the Sun, but anyway, on the same line.

But the lifting of the water, the bulges of the tides, are not really created directly by the lifting from the Moon attraction, because this force is really, really small and can not lift water. The bulge is created by the resultant forces of every small unit of water who feel this force, especially the tangent ones, and who push the water in the oceans in the direction of the Moon. Because the water is fluid but incompressible, the sum of this myriad units create the whole bulge.



That's why you don't have tides in lakes or small seas. Because is not enough water to push a bulge.

The opposite side of the bulge is more tricky. Because the force of gravity decrease with the square of distance, the Earth center will be much closer to the moon than the opposite side. So the opposite side will feel from the Moon a force much weaker than the Earth center and muuuch more weak than the point below Moon. You really can imagine that the Earth accelerate, fell towards the Moon and the water is left behind.

And forget the theory of centrifugal force explanation. The centrifugal force is very, very weak in this case.

A fun example easy to understand the forces involved of Moon and Sun: Assuming you have a weight of 70kg, then, when Moon is up at zenith, you will be lighter (attracted by the Moon) with about  1.65∗10−4  kg, or the equivalent weight of 10 flies. But when the Sun is at zenith, you are slightly lighter with only about  9.7∗10−7  kg or the equivalent weight of an eyelash, Maximum difference will be on New Moon when the Sun and Moon are on the same side above you and their force of gravity combine to pull you from Earth, especially in December or January when Sun is closer. Also then, in spring, are the greater tides.

"Gravity and inertia act in opposition on the Earth’s oceans, creating tidal bulges on opposite sites of the planet. On the “near” side of the Earth (the side facing the moon), the gravitational force of the moon pulls the ocean’s waters toward it, creating one bulge. On the far side of the Earth, inertia dominates, creating a second bulge."



https://oceanservice.noaa.gov/education/tutorial_tides/tides03_gravity.html

I mainly focus on resultant of gravitational force among Sun and Moon on Earth, using tide as measurement seems not suitable, since Gravity and inertia act in opposition on the Earth’s oceans, if we look at physics alone, would it be possible to list out the following resultant gravitational force among Sun and Moon on Earth from strongest to weakest orders?



1) New Moon
2) Waxing Crescent
3) First Quarter
4) Waxing Gibbous
5) Full Moon
6) Waning Gibbous
7) Third Quarter
8) Waning Crescent

Do you have any suggestions?
Thank you very much for any suggestions (^v^)

Hi oemBiology

The calculations for these are actually quite complex but doable.

When the Moon is in the gibbous phase for example, it is not aligned with the Earth and Sun, but is rather at an angle. Therefore, you cannot simply add up the gravitational forces between the three objects to get the net gravitational force. Instead, you need to use vector addition to determine the direction and magnitude of the net force.

Let's look at this from a 2D perspective. Let's say we want to calculate the net force on the earth when the moon is at 45 degrees. To calculate the net gravitational force on the Earth when the moon is at 45 degrees from the Earth, we need to consider the gravitational forces of both the Moon and the Sun.

Let's start by calculating the gravitational force of the Moon on the Earth:

The formula for the gravitational force between two objects is:

F = G * (m1 * m2) / r^2

where F is the force, G is the gravitational constant, m1 and m2 are the masses of the two objects, and r is the distance between them.

The mass of the Moon is 7.34 x 10^22 kg, and the mass of the Earth is 5.97 x 10^24 kg. The distance between the Earth and the Moon varies depending on their positions in their orbits, but at a distance of 45 degrees, it is approximately 3.63 x 10^8 meters.

Plugging these values into the formula, we get:

Fmoon = G * (m1 * m2) / r^2
= 6.67 x 10^-11 * (7.34 x 10^22 * 5.97 x 10^24) / (3.63 x 10^8)^2
= 1.99 x 10^20 N

This is the gravitational force of the Moon on the Earth when the Moon is at 45 degrees from the Earth. Note that this force is directed towards the Moon.

Now, let's calculate the gravitational force of the Sun on the Earth:

The mass of the Sun is 1.99 x 10^30 kg, and the distance between the Earth and the Sun is approximately 1.50 x 10^11 meters.

Using the same formula as before, we get:

Fsun = G * (m1 * m2) / r^2
= 6.67 x 10^-11 * (1.99 x 10^30 * 5.97 x 10^24) / (1.50 x 10^11)^2
= 3.52 x 10^22 N

This is the gravitational force of the Sun on the Earth. Note that this force is directed towards the Sun.

To calculate the net gravitational force on the Earth, we need to add the gravitational forces of the Moon and the Sun vectorially, taking into account their directions.



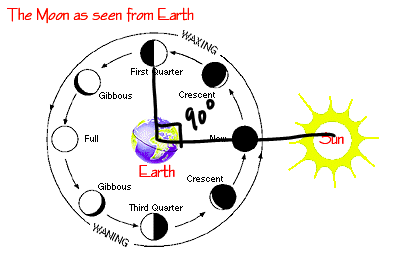
If we draw a diagram, we can see that the gravitational force of the Moon is at an angle of 45 degrees to the gravitational force of the Sun, and the direction of the net force is between the two forces.

Using trigonometry, we can find the magnitude of the net force:

Fnet = sqrt(Fmoon^2 + Fsun^2 + 2 * Fmoon * Fsun * cos(45))
= sqrt((1.99 x 10^20)^2 + (3.52 x 10^22)^2 + 2 * (1.99 x 10^20) * (3.52 x 10^22) * cos(45))
= 3.52 x 10^22 N

Therefore, the net gravitational force on the Earth when the Moon is at 45 degrees from the Earth, taking into account the gravitational force of the Sun, is approximately 3.52 x 10^22 N.

This is a very general calculation, but if you were doing this more specifically, you need to determine the components of each gravitational force in the x, y, and z directions, and then add up the components separately. The calculations involved are quite complex and involve multiple variables that vary depending on the Moon's position.

Hope that helps

Would it be possible to list out all distance at different angles in order to calculate the resultant force?

Full moon supposes to be the lowest resultant force, but based on your calculation is not.
I simply replace 45 with different angle, and come out with following results.

Fnet = sqrt(Fmoon^2 + Fsun^2 + 2 * Fmoon * Fsun * cos(45))
= sqrt((1.99 x 10^20)^2 + (3.52 x 10^22)^2 + 2 * (1.99 x 10^20) * (3.52 x 10^22) * cos(45))
= 3.52 x 10^22 N



Do you have any suggestions?
Thank you very much for any suggestions (^v^)

What number did you replace within cos(?) ? In a full moon, you don't need to use components in your calculation (as I did here), since the vectors are on the same plane.

I replace numbers as shown below

Cos(180 degree) should be -1, but if I simply input Cos(180) would get -0.6, so it must be sometimes wrong for using Cos here.

0   3.54E+22
45   3.53E+22
90   3.51E+22
135   3.50E+22
180 3.51E+22
225 3.53E+22
270 3.54E+22
315   3.53E+22

Do you have any suggestions?
Thanks, to everyone very much for any suggestions (^v^)

Post Merge: 2 months ago

I replace numbers as shown below, it seems that Full moons get the lowest resultant of gravitational force, correct?

= sqrt((1.99 x 10^20)^2 + (3.52 x 10^22)^2 + 2 * (1.99 x 10^20) * (3.52 x 10^22) * cos(PI()/180*45))
= 3.534E+22 N

0   3.540E+22 N
45   3.534E+22 N
90   3.520E+22 N
135   3.506E+22 N
180 3.500E+22 N
225 3.506E+22 N
270 3.520E+22 N
315   3.534E+22 N



Do you have any suggestions?
Thanks, to everyone very much for any suggestions (^v^)

That is correct. Here's also another handwritten sample calculation

Thanks, to everyone very much for suggestions (^v^)

I forgot to add the first part of the calculation, sorry.